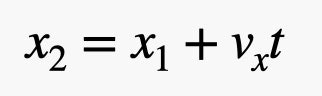The time it takes for Drake to move in the vertical direction is the exact same time it takes for him to move horizontally. This means that I can use the horizontal motion to calculate the time, and then use that amount of time in the vertical motion to find his final vertical position.
When Drake makes his jump, he needs to get up to a vertical position of zero meters; that’s the position of the ramp and where I set the origin. If this final value is less than zero meters, he lands below the airplane. And that would be bad.
Determining the horizontal motion isn’t too difficult. Since he has a constant velocity, I can find his final horizontal position with the following equation:
Illustration: Rhett Allain
Check this out: I know the starting x-position (x1 = 2.4 m) and the final x-position (x2 = 0 m) so that I can use the x-velocity to solve for the time it takes to complete the jump. (He’s moving to the left, so that’s going to be negative 3.37 m/s.)
Notice that in the trailer we don’t see the whole jump, but, if we did, it would take 0.71 seconds to reach the back ramp of the aircraft.
Now, I can use this time and plug it into the vertical kinematic equation. This gives a final y-position of negative 1.79 meters.
That’s lower than zero, so there’s nothing but air below him. And remember: That’s bad.
We’re not done yet, but it’s worth taking a second to wonder why he ends up even lower than he started. It’s because even though his initial velocity is in the positive (upward) direction, the jump takes so long that the gravitational force stops his upward motion and makes him move downward at a faster and faster rate.
What About the Moving Air?
When you stick your hand out the window of a moving car, you can feel something pushing back on you. This is the interaction between your hand and the air molecules surrounding the car—we call this air resistance. The amount of force you feel depends on the relative speed of the hand with respect to the air, and the size and shape of your hand. At very large speeds, this air resistance force can be significant.
Let’s say the aircraft has a flying speed of 120 mph—I like that value because it’s the same as the terminal velocity of a human skydiver. When someone falls through the air for a while, the gravitational force causes them to increase in speed. But this increase in velocity also increases the upwards-pushing air resistance. At some point not too long after a jump, the upward air resistance force is equal to the downward gravitational force. This means the total force is zero and the diver no longer accelerates. Instead, now they move at a constant speed. We call that the terminal velocity. Of course, humans can still adjust their body and interact with the air to turn and maneuver—that’s why skydiving is still fun.