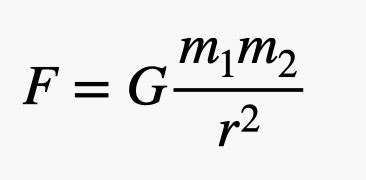It’s fun to think about how we know stuff. For instance, the sun has a mass of about 2 x 10 30 kilograms. That is such an enormous mass that it’s difficult to comprehend. And if it’s so difficult for us to even imagine numbers that large, how would we go about finding those values? Well, the original method was to use some small masses, a stick, and a string. Yes, this is one of the important steps in determining masses of both the sun and all the planets in our solar system. It’s called the Cavendish experiment—first performed by Henry Cavendish in 1798. It’s really cool, so I’m going to explain how it works.
Objects with mass have a gravitational attraction between them. A basketball has a gravitational interaction with Earth (since they both have mass). It is this gravitational interaction that makes the basketball speed up as it falls toward the ground if you let go of it. But of course everyone has always known that if you let go of an object it will fall. However, it was around the time of Newton that people realized that this interaction also worked with astronomical objects like Earth, moon, and sun. That gives us this force model—it’s often called Newton’s Law of Universal Gravity, but like most big ideas it likely had a lot of contributors.
Illustration: Rhett Allain
Let’s go over this gravitational force model. First, the magnitude of this force depends on the product of the two masses interacting (m1 and m2). Second, the magnitude decreases with the square of the distance between the two objects (r). Finally, there’s that G. This is the universal gravitational constant. It’s the key to finding the mass of Earth.
So, just step back for a moment. When we measure stuff, we always have to make some type of choice. If we want to have a mass in kilograms, then we have to decide how to specify the value of 1 kg. One way would be to say that a kilogram is the mass of 1 liter of water. Of course, that’s not the best definition (we have better methods now). OK, what about measuring force? We use a unit called the Newton where 1 Newton is the force required to accelerate 1 kilogram at 1 meter per second per second. Yes, things are getting out of control—but the key is that you can make these definitions and build one unit on another unit.