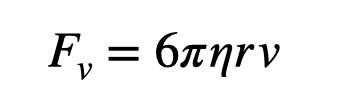Right now, you can probably just ask your smartphone to tell you the charge of a single electron—the fundamental unit of charge. (It has a magnitude of 1.6 x 10–19 coulombs, the common unit for electric charge.) But in 1909, things weren’t so simple. Back then, physicists Robert Millikan and Harvey Fletcher figured it out using oil. Their “oil drop” experiment wasn’t the first method to find this value, but it’s perhaps the most famous, and it led to Millikan receiving the Nobel Prize in 1923.
This historic experiment illustrates some important physics concepts, and it’s not too terribly complicated, so let’s go over them!
The Four Forces
This experiment deals with oil drops—I mean, it’s right there in the name. But, really, it depends on understanding four different forces: the gravitational force, the electric force, the buoyancy force, and an air resistance force. The idea is to use these four to measure the value of the electric charge on a single drop of oil.
Surely, you already know about the gravitational force. If I had to guess, I would say that you are somewhere on the surface of the Earth. That means you are probably experiencing a gravitational force as an interaction between your mass and the Earth’s mass. We can model this interaction by considering the Earth as creating a gravitational field—a downward-pointing vector with a magnitude of 9.8 newtons per kilogram. A mass in this gravitational field will experience a force equal to the product of the object’s mass and the gravitational field. (Of course, this is just a model. If you move too high above the Earth, you will need a different model.)
The next one is the electric force. This is an interaction between any two objects that have electric charge. Just like with the gravitational force, we can find the electric force by putting a single charge in a region with an electric field (E) in units of newtons per coulomb. The electric force will then be the product of the object’s charge (q) and the electric field.
The previous two forces seem to complement each other. But the next two are a bit different. They have to do with the interaction between the oil and the air it is falling through. You already understand the air drag force if you’ve ever stuck your hand out of the window of a moving car. As you increase the speed of the car, this air drag force on your hand also increases.
For objects the size of your hand, the air drag force is proportional to the square of the hand’s velocity. However, if you have a very small spherical object (like a drop of oil) moving through the air, we can model this force with the following equation:
Illustration: Rhett Allain